Properties Of Log What Are Logarithmic Properties
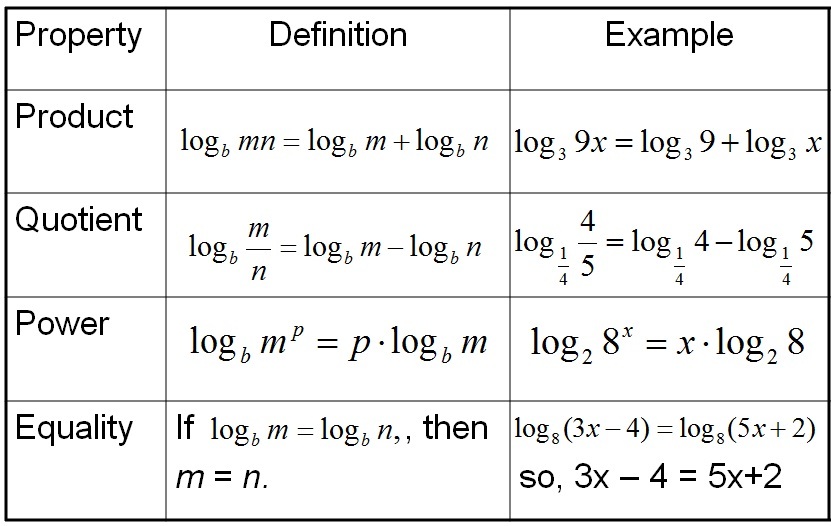
Logarithmic Laws And Properties The logarithmic properties are applicable for a log with any base. i.e., they are applicable for log, ln, (or) for logₐ. the 3 important properties of logarithms are: log mn = log m log n log (m n) = log m log n log m n = n log m; log 1 = 0 irrespective of the base. logarithmic properties are used to expand or compress logarithms. Logarithm base properties. before we proceed ahead for logarithm properties, we need to revise the law of exponents, so that we can compare the properties. for exponents, the laws are: product rule: a m.a n =a m n. quotient rule: a m a n = a m n. power of a power: (a m) n = a mn. now let us learn the properties of logarithmic functions.
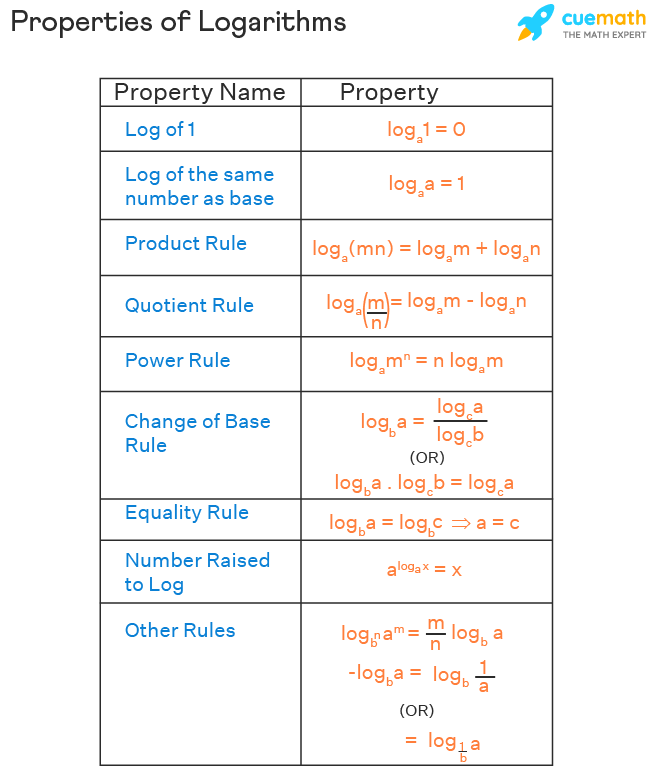
Properties Of Log What Are Logarithmic Properties Key takeaways. given any base b> 0 and b ≠ 1, we can say that logb1 = 0, logbb = 1, log1 bb = − 1 and that logb(1 b) = − 1. the inverse properties of the logarithm are logbbx = x and blogbx = x where x> 0. the product property of the logarithm allows us to write a product as a sum: logb(xy) = logbx logby. The logarithm properties are: the logarithm of a product is the sum of the logarithms of the factors. log a xy = log a x log a y. the logarithm of a quotient is the logarithm of the numerator minus the logarithm of the denominator. log a = log a x log a y. log a x n = nlog a x. where x and y are positive, and a > 0, a ≠ 1. Surprisingly though, the four properties of logarithm involving the bases (i.e., change of base rule, chain rule, base swapping rule, base argument interchangeability) will be all preserved. due to the substantial loss in the properties of logarithm, most of the properties of exponent will be falling apart as well. Notice, we had to rewrite \(2 \log 2 x\) as \(\log 2 x^2\) in order to see there was a power on \(x\) in which we had to use the product property of logarithms to write \(2\) as the exponent. thus, all factors are written as powers, all sums are written as products, and all differences are written as quotients.
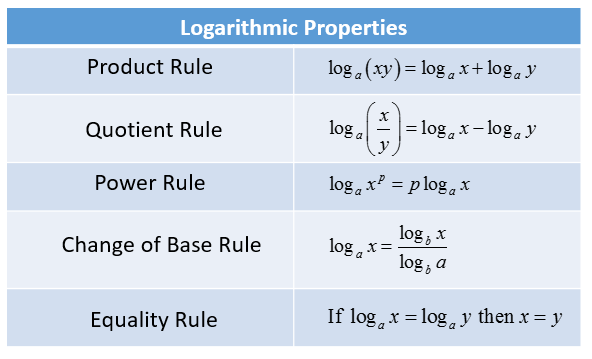
Properties Of Logarithms Worksheet Pdf Surprisingly though, the four properties of logarithm involving the bases (i.e., change of base rule, chain rule, base swapping rule, base argument interchangeability) will be all preserved. due to the substantial loss in the properties of logarithm, most of the properties of exponent will be falling apart as well. Notice, we had to rewrite \(2 \log 2 x\) as \(\log 2 x^2\) in order to see there was a power on \(x\) in which we had to use the product property of logarithms to write \(2\) as the exponent. thus, all factors are written as powers, all sums are written as products, and all differences are written as quotients. Given a sum, difference, or product of logarithms with the same base, write an equivalent expression as a single logarithm. apply the power property first. identify terms that are products of factors and a logarithm, and rewrite each as the logarithm of a power. next apply the product property. rewrite sums of logarithms as the logarithm of a. The opposite of expanding a logarithm is to condense a sum or difference of logarithms that have the same base into a single logarithm. we again use the properties of logarithms to help us, but in reverse.

Understanding The Properties Of Log Functions Mathodics Given a sum, difference, or product of logarithms with the same base, write an equivalent expression as a single logarithm. apply the power property first. identify terms that are products of factors and a logarithm, and rewrite each as the logarithm of a power. next apply the product property. rewrite sums of logarithms as the logarithm of a. The opposite of expanding a logarithm is to condense a sum or difference of logarithms that have the same base into a single logarithm. we again use the properties of logarithms to help us, but in reverse.
Comments are closed.